Since introduction of zirconia as a restorative core material, zirconia (zirconium dioxide) has radically increased the use of ceramic restorations in dentistry. CAD/CAM technology has contributed tremendously to make this possible. Unique qualities of zirconia like its high strength, superior mechanical properties, aesthetic colour, chemical stability, made it the most favoured core material [1]. But, zirconia lacks the translucency of natural teeth. Therefore, to achieve greater aesthetics, zirconia cores or frameworks must be veneered with weaker porcelain [2]. Clinical studies of zirconia-based all-ceramic restorations have shown encouraging clinical results with high survival rates. Yet, chipping, fracture and delamination of the ceramic veneer is the major trouble in such restorations with a reported incidence between 0 and 30% [3]. To utilise the strength of the core material, the core-veneer bond strength must be strong enough to transmit functional stresses from the aesthetic veneer to the underlying framework. However, according to Aboushelib, the zirconia-veneer bond strength was inferior compared to other all-ceramic systems. Thus, low core-veneer bond strength can result in chipping and/or delamination of the veneer ceramic [1]. Also, there are no clear data with respect to the bond stability between zirconia and ceramic veneers under loads [4]. Focus on the factors like framework design can improve clinical performances as it is an important factor which is technique-sensitive and can easily influence the failure of final restorations [5]. Hence, this study was undertaken to investigate how the design characteristics of the junction between ceramic and zirconia affect the mechanical behaviour of ceramic layered zirconia restorations in relation to three different designs of zirconia-ceramic veneer interface, under various loading conditions, with the use of a three-dimensional finite element analysis.
Materials and Methods
Three patients suggested for zirconia based ceramic restoration in mandibular molars, who had visited the Department of Prosthodontics, Crown and Bridge, Krishnadevaraya College of Dental Sciences and Hospital, Bengaluru, India, were selected. Patients were informed about the procedure in detail and informed consent form was signed by them. An in vivo study was conducted for two years which was approved by the Ethical Committee of Krishnadevaraya College of Dental Sciences and Hospital, Bengaluru, India.
Tooth preparation of the indicated mandibular molar was done for the three patients. Impressions were made using putty relining technique with the help of putty and light body polyvinylsiloxane material (Dentsply) and the models were obtained (Kalabhai Type IV gypsum product). Models were scanned in the model scanner and with the help of CAD/CAM milling unit, zirconia (Amann Girrbach, ceramill ZI) framework of various designs were milled on which ceramic veneer (IPS E.max Ceram, Ivoclar) layering was done manually according to the particular design characteristic. Thus, each patient was restored with a different design of zirconia-ceramic interface. CBCT image was taken after the cementation of the zirconia based ceramic restoration.
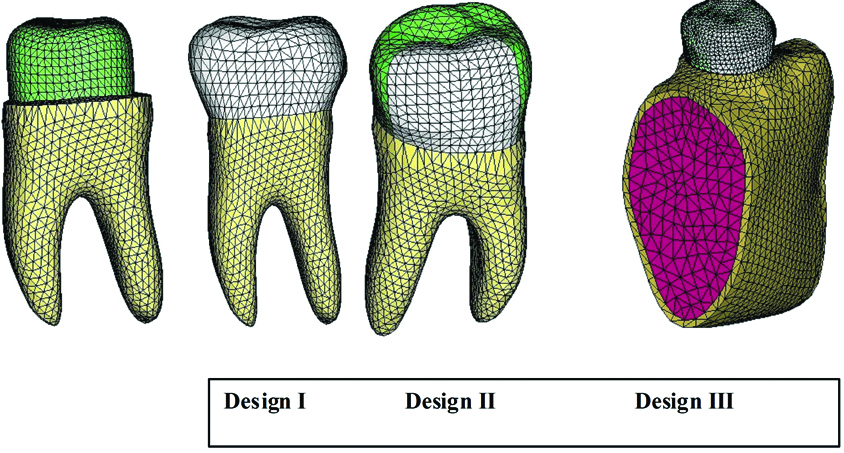
The number of crowns representing each design was decided to be one in number and a CBCT image of the same was taken for developing the finite element models. Schematic diagrams of the three different designs of zirconia-ceramic interface are shown in [Table/Fig-1,2 and 3]. Slices of the CBCT image were serially selected and processed by an interactive image control system (Mimics) for three-dimensional reconstruction. The software presents segmentation functions based on image density thresholds [6]. Identical geometrical models incorporating the various designs were developed by applying Rapidform (V2004) software that includes all the surface data. The 3D object representing the zirconia framework and ceramic veneer were obtained. This model was meshed by applying Hypermesh (13.0) software to achieve a Finite Element Model (FEM) [Table/Fig-4]. Each FEM consists of numerous nodes and tetrahedral elements. The models had 98112 (design I), 96048 (design II), 98387 (design III) elements. The number of nodes were 18628 (design I), 17573 (design II), 18145 (design III). All the tooth structure (enamel, crown and root dentin, dental pulp, periodontal ligament, soft and hard bone) were included in the solid model. The model had a homogeneous tooth reduction of 1.5 mm on the buccal and lingual surfaces and 2.0 mm on the occlusal surface. Accordingly, the ceramic veneer thickness was 1.0 mm on the buccal and lingual surfaces and 1.5 mm on the occlusal surface and zirconia core thickness of 0.5 mm.
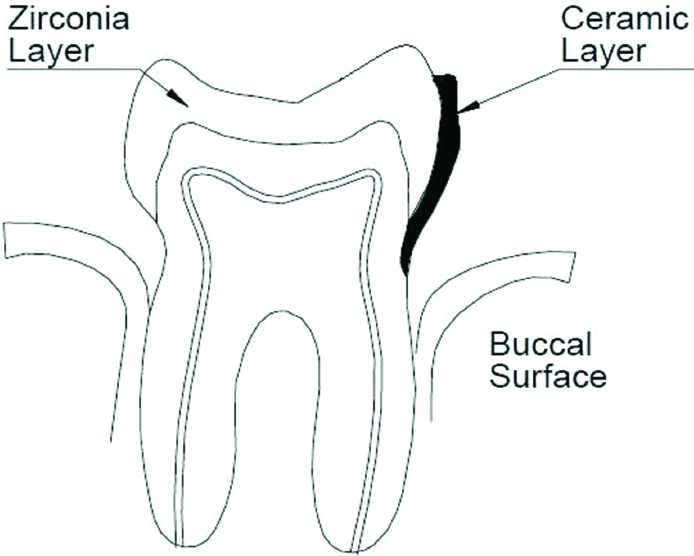
Design I of zirconia-ceramic interface.

Design II of zirconia-ceramic interface.
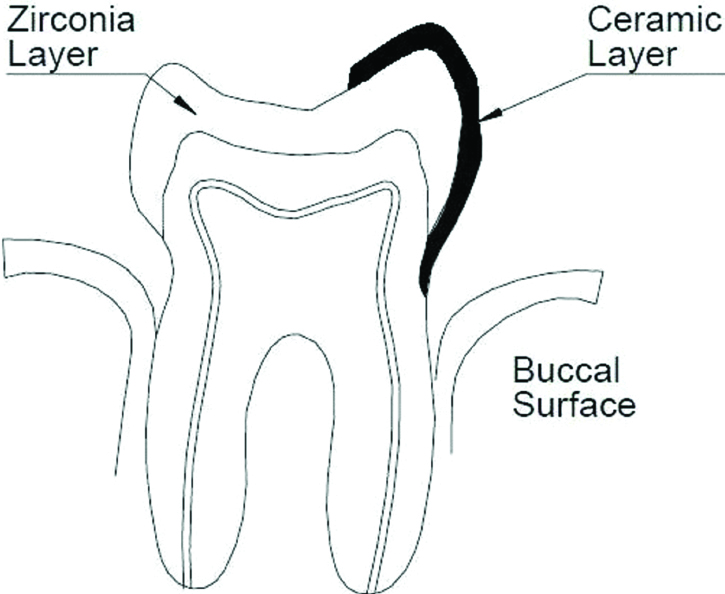
Design III of zirconia-ceramic interface.
Finite Element Models for design I, II, III.
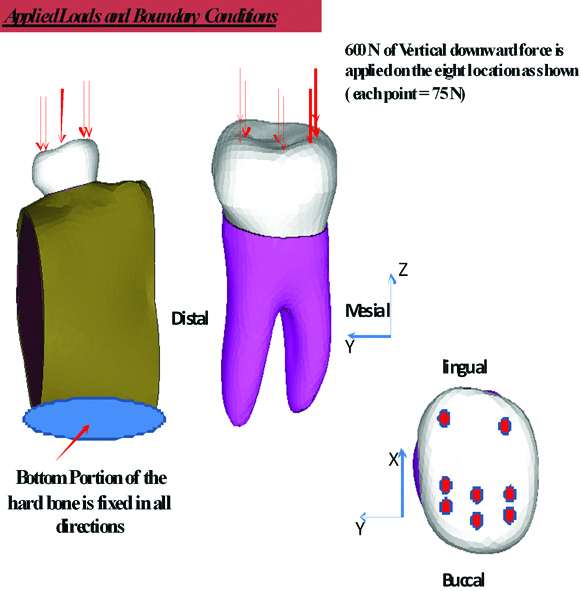
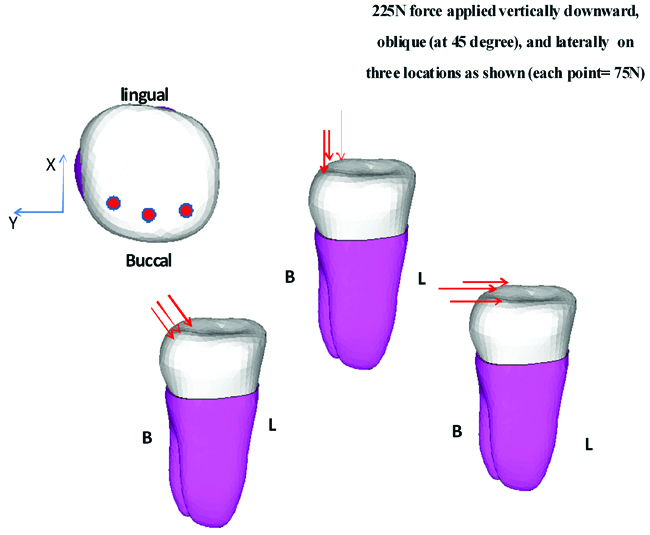
Four types of loads were applied to each of the three finite element model under boundary conditions [Table/Fig-5,6].
Loading points and directions simulating maximum bite force. Eight loading points: three points on the outer inclines of the buccal cusps, three points on the inner inclines of the buccal cusps, and two points on the inner inclines of the lingual cusps. A total load of 600 N was applied from the axial (vertical) direction.
Loading points and directions simulating masticatory forces. Three points were loaded on the outer incline of the buccal cusps. The total load of 225 N was applied from three directions.
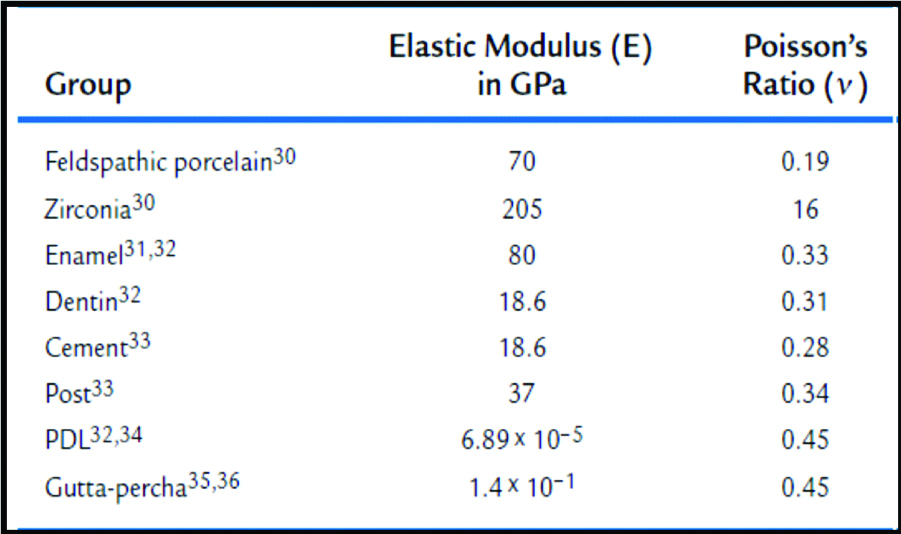
The following considerations were made to facilitate the FEA analyses: 1) the materials were considered to be isotropic and homogeneous and to have a linear elastic behaviour; 2) the effects of the periodontal ligament and pulp chamber were not considered; 3) elastic modulus 4); and Poisson’s ratio; 5) were maintained constant [6]. The values of the material properties used to perform the analysis are presented in [Table/Fig-7] [4]. Volume meshes were exported to the FEA software (ANSYS 12.1), and the load-generated stress distribution was analysed numerically. The maximum principal stress (σmax) and minimum principal stress (σmin) were obtained for zirconia and ceramic veneer. The readings were tabulated and graphically represented. According to Misra A et al., maximum principal stress (σmax) is appropriate for predicting failures in nonductile materials [7], such as ceramics, as well as failures that could begin from small flaws [4]. There was no statistical analysis required for this study and the results were interpreted by the FEA software (ANSYS 12.1) numerically.
Mechanical properties of the materials.
Results
Maximum principal stress criterion states that failure will occur when maximum principal stress developed in a body exceeds the ultimate tensile strength of the material. The maximum principal stress is the most tensile (least compressive) and the minimum principal stress is the least tensile (most compressive) in nature. The ultimate tensile strength of zirconia is around 1200 MPa [8]. The tensile strength is approximately 29 MPa for opaque feldspathic porcelain and the compressive strength is reported to be 340 MPa for a feldspathic porcelain [9].
Value of Maximum principal stress (tensile stress) generated with each force in each design was recorded and tabulated for ceramic layer and zirconia layer. These values were compared amongst the three designs with the help of graphical representations.
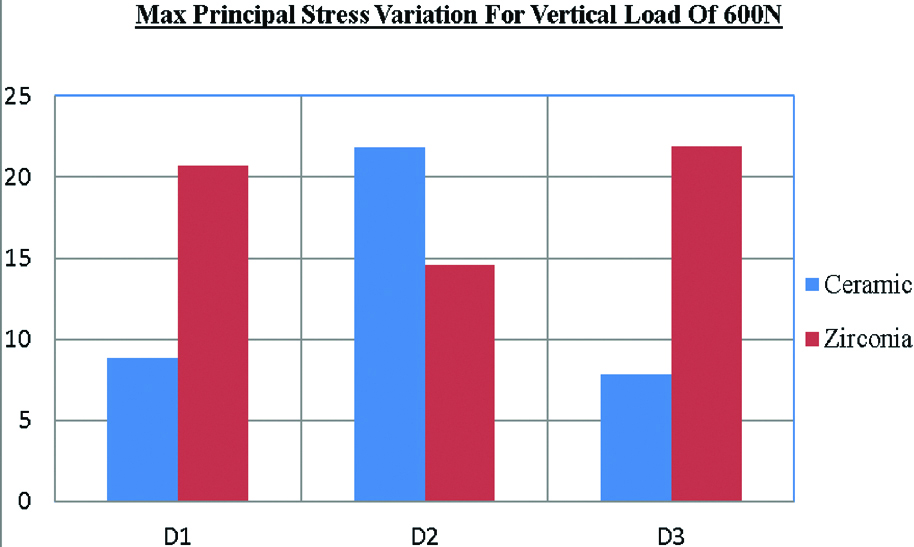
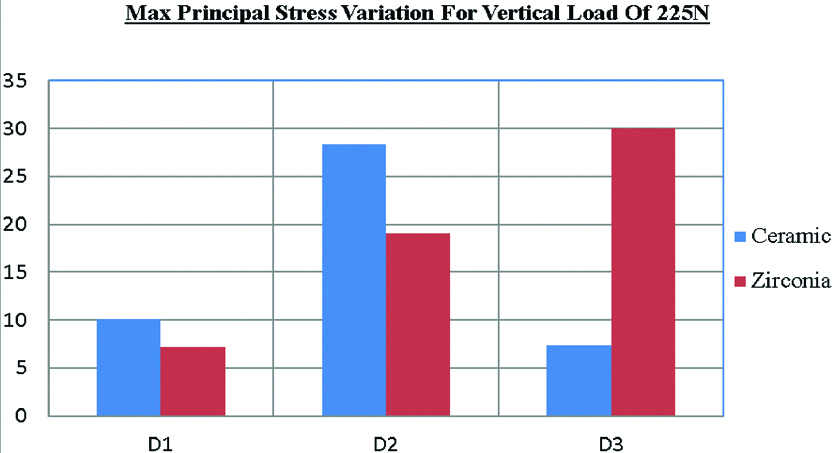
When vertical loads of 600 N and 225 N were applied on all the three designs, ceramic layer of design II experienced larger tensile stresses 21.83 MPa and 28.33 MPa respectively in comparison to other two designs which observed tensile stresses of less than 10 MPa. While, in zirconia layer larger tensile stresses were observed in design III, 21.9 MPa and 30 MPa respectively for vertical load of 600 N and 225 N [Table/Fig-8,9].
Unit of y axis- Mega Pascal (MPa).
D1: Design I; D2: Design II; D3: Design III.
Unit of y axis- Mega Pascal (MPa).
D1: Design I; D2: Design II; D3: Design III.
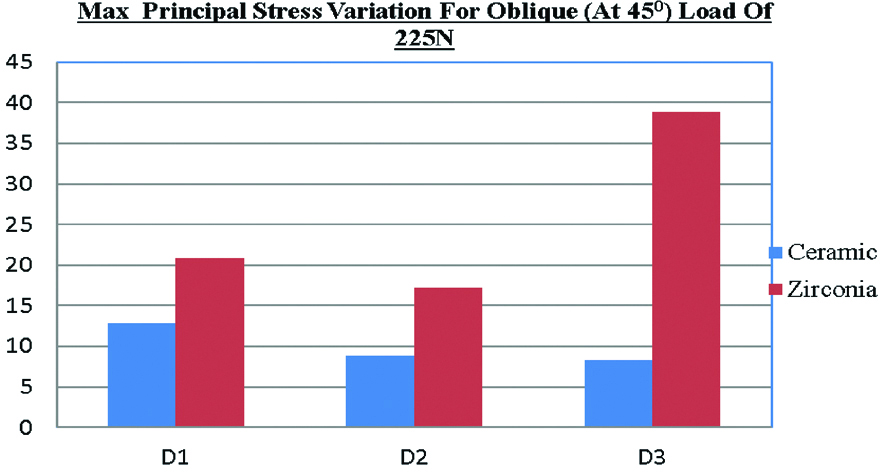
When an oblique load (at 45° angle) of 225 N was applied, largest tensile stress in ceramic layer (12.82 MPa) was observed in design I in comparison to other designs which observed tensile stresses less than 9 MPa [Table/Fig-10]. While maximum tensile stress in zirconia layer (38.92 MPa) was experienced in design III [Table/Fig-10]. The resultant tensile stress was less when the load was applied at a 45° angle than when it was applied vertically or horizontally.
Unit of y axis- Mega Pascal (MPa).
D1: Design I; D2: Design II; D3: Design III.
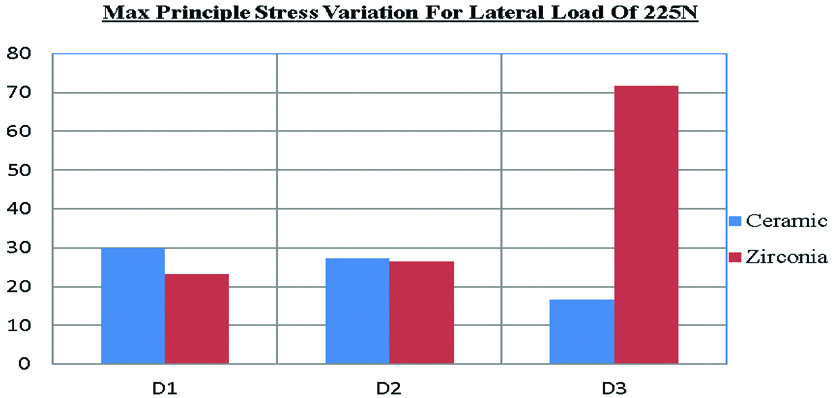
When horizontal load of 225 N was applied, tensile stress in ceramic layer was largest in design I i.e., 29.87 MPa. The tensile stress of design II was 27.23 MPa which is almost comparable to design I. Design III experienced much lesser tensile stress of 16.65 MPa [Table/Fig-11]. While in zirconia layer, largest tensile stress (71.74 MPa) was experienced in design III [Table/Fig-11]. In all the three designs, ceramic layer experiences highest tensile stress when subjected to horizontal forces. Hence, from the simulation results and graphs it is evident that horizontal (lateral) forces are of concern for ceramic layer.
Unit of y axis- Mega Pascal (MPa).
D1: Design I; D2: Design II; D3: Design III.
Discussion
Inspite of being the most stable framework material amongst all the dental ceramics, zirconia frequently displays a technical problem of minor chipping or extended fracture of the ceramic veneer in zirconia based all ceramic restorations. An insufficient bond between the veneering ceramic and the zirconia framework is of major concern. In a prospective three year study, ceramic chipping was reported in about 13% of the zirconia fixed dental prostheses observed. Many variables, either isolated or combined, such as framework design, the surface finish of the core, residual stresses generated by a mismatch in the Thermal Expansion Coefficients (TECs) of the ceramic and zirconia structures, the development of flaws and defects at the core/veneer interface, as well as wetting properties and volumetric shrinkage of the veneer may affect the zirconia core/veneer bond strength and, consequently, the clinical success rate of the restorations [4]. Optimum thickness of the ceramic core and the veneering porcelain ensures that the veneering porcelain is under compressive stress and that the ceramic core is under tensile stress which helps in controlling the crack initiation site and potential failure. Though, the veneering porcelain is used primarily for aesthetic reasons, it also significantly affects the mechanical behaviour of the restoration. Flexural strength of the bilayered restoration is increased by the residual compressive stresses in the veneer layer but, chipping is primarily caused by the tensile stresses. High elastic modulus of zirconia framework reduces the stress on the weaker veneer layer and increases the composite load-bearing capacity, thereby retarding the fracture of the restoration. Following traditional preparation guidelines help in stress distribution during dynamic loading of the restoration [9].
The zirconia used as a core material for this study is yttria-stabilized zirconia (Amann Girrbach, ceramill ZI) and feldspathic porcelain used for veneering (IPS E-max ceram, Ivoclar) with coefficient of thermal expansion of the core material slightly greater than that of the veneering ceramic. This slight mismatch in CTE causes residual compression in the porcelain veneer layer and provides additional strength.
Finite Element Analysis (FEA) is a fast and a relatively low cost method used to investigate stress distribution and strain patterns of complex structures, such as dental restorations. FEA can be used to understand the failure behaviour of complex structures, or to optimize the experiments through the mathematical simulation and selection of the best design to perform the test.
Usual masticatory force is reported to range from approximately 37 (Lundgren D and Laurell L, in 1986) to 40% (Gibbs CH et al., in 1981) of the maximum bite force [10,11]. On the basis of this report, a load of 225 N, which is 37% of the maximum biting force of 600 N, was applied from three directions [12]. In a study of healthy people, it was reported that the masticatory force on the lower first molar is applied at 20-280 to the tooth axis (Morikawa A, in 1994) [13].
Taskonak B et al., established in their study that failure stresses of the fixed partial dentures that failed due to zirconia fracture ranged from 379 to 501 MPa [14]. Fractures that had origins on the glass veneer surface had failure stresses between 31 MPa and 38 MPa. In the present study, the maximum tensile stresses which were produced in zirconia layer when various loads were applied [Table/Fig-12], are substantially less than the failure stresses for zirconia reported in the above literature (379-501 MPa). Also, zirconia has high tensile and flexural strength which ranges from 900-1200 MPa and high modulus of elasticity of upto 200 GPa with unique property of transformation toughening. Thus, zirconia cores are able to withstand the high occlusal stresses occurring during function. But, the strength of a non-homogenous (layered) all ceramic structure is determined by its weakest component which is usually the core-veneer bond strength or the veneering material itself, which has to be strong enough to withstand the stresses of mastication to prevent delamination and fracture of the veneering material. However, according to Aboushelib, zirconia core-ceramic veneer bond strength is lower than for other all-ceramic systems. He evaluated the microtensile strength and bond strength of a variety of widely used core and veneering ceramics and found that despite the high microtensile strength (340 MPa) of zirconia, its bond strength with veneering ceramic was 29 MPa. The MTBS test has proven to be a reliable test for evaluating the bond strength of composite materials to a variety of substrates [15].
Max principal stress in ceramic layer and zirconia layer.
| Vertical 600 N | Vertical 225 N | Oblique 225 N | Lateral 225 N |
|---|
| Ceramic | Zirconia | Ceramic | Zirconia | Ceramic | Zirconia | Ceramic | Zirconia |
| Design I | 8.83 MPa | 20.7 MPa | 10.07 MPa | 7.19 MPa | 12.82 MPa | 20.96 MPa | 29.87 MPa | 23.19 MPa |
| Design II | 21.83 MPa | 14.6 MPa | 28.33 MPa | 19.1 MPa | 8.88 MPa | 17.25 MPa | 27.23 MPa | 26.39 MPa |
| Design III | 7.87 MPa | 21.9 MPa | 7.41 MPa | 30 MPa | 8.32 MPa | 38.92 MPa | 16.65 MPa | 71.74 MPa |
Fracture has a typical evolution in a bilayered ceramic: a superficial layer, the ceramic veneer, is not as tough as the deeper zirconia core layer, and these two layers are linked by a weak bonding mechanism. In this situation, when catastrophic fracture occurs, delamination is common. This occurs because cracks are unlikely to propagate from a ceramic with a low modulus of elasticity and a low level of toughness to a ceramic like zirconia with a high modulus of elasticity and greater toughness [4].
On application of lateral load, tensile stresses in ceramic layer of design I and II [Table/Fig-12,13] are almost equal to the previously reported failure stress of ceramic layer by fractographic analysis. Also, the tensile strength, as determined by diametral compression, is approximately 29 MPa for opaque feldspathic porcelain [9]. Thus, there are chances of chipping of ceramic veneer in design I and II on application of lateral load. Bite forces applied from the horizontal direction are a critical factor. But there is little likelihood of such a strong masticatory force being applied horizontally during usual mastication. It has been reported, however, that in some cases patients with bruxism or clenching exert a supra-physiological force (i.e., a force greater than the maximum bite force of healthy people) on their teeth during tooth-grinding. Such patients might face the possibility of a fractured crown [10]. On application of vertical load of 225 N, tensile stress in ceramic layer of design II [Table/Fig-12,14] is almost equal to the tensile strength for feldspathic porcelain, but result is not similar when vertical load of 600 N is applied. This may be because of the difference in loading points in both the cases.
Lateral load of 225 N on ceramic layer in design I, σmax=29.87 MPa.
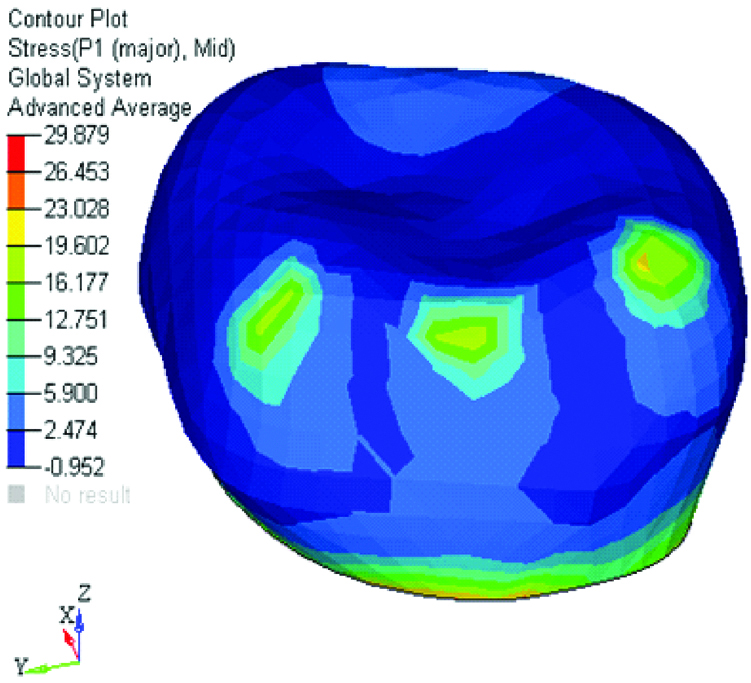
Vertical load of 225 N on ceramic layer in design II, σmax=28.33 MP.

Limitation
Zirconia veneer bond strength is sensitive to many interacting variables, such as the type of veneer ceramic and its method of application and surface preparation of zirconia framework [16]. In the present study, only one type of zirconia core material and feldspathic porcelain veneer material has been used. A clinical study can follow this finite element analysis to rationalize the obtained results and to evaluate the prognosis of the restoration. Further studies are required to achieve a gold standard for zirconia-ceramic veneer bond strength.
Conclusion
The different design characteristics of the junction between ceramic and zirconia will affect the bond between zirconia core and ceramic veneer. Zirconia crowns with ceramic veneer only on buccal surface have the least chances of chipping of ceramic layer under masticatory loads. Thus, this design should be preferred in posterior restorations such as in molars due to higher masticatory forces present in this region and in patients suffering from bruxism. Zirconia crowns with ceramic veneer on buccal surface extending upto the occlusal surface is more prone to chipping of ceramic layer under masticatory loads. Zirconia crowns with full ceramic veneer may show chipping under high occlusal loads. In cases where aesthetics is paramount, like premolars or smile designing, full veneer zirconia restorations are indicated.